A notable coffee roasting consultant recently wrote an article suggesting that coffee roasters shouldn't sell bags of blended coffee... that they should sell separate bags of the component coffees of their blends and provide the recipe ratios for their customers to correctly mix their blends. The contention was that each time a customer made their coffee that the blend in the bag might not represent the blend recipe closely enough to express the creative intent of the coffee roaster. This struck me as an extraordinary suggestion.
Those of you who know me understand that I'm a glutton for punishment... I really do like to make life difficult. I drive a manual car (yes my left foot frequently depresses a clutch pedal), I went to university for a total of eight years (no I didn't fail any years), and we have the most energetic golden retriever as a pet (all 35 kilograms of her). Furthermore, I do weigh each dose of coffee that I grind and also weigh the espresso output. I'm more than happy to make work for myself. I also realise that I'm on the crazy end of the spectrum. Do most home coffee makers want to be weighing out multiple doses of component coffees to exactly replicate a hypothetical ideal blend? Could most people taste the difference if the blend were out by a few percent?
I decided to think about this question mathematically. It was good to be able to chat to someone with strong mathematical background (thank you Murray) to set me off on the right path. The thought experiment models a simple blend of two beans blended 50/50. I'm making the following assumptions: that the two different beans have the same density and same volume; that each bean weighs 0.1 grams; that the bag of coffee blend has the right proportions overall as a starting point (i.e. 50/50); that the bag is large enough that I can assume that with each bean coming out of the bag that I don't need to make minute adjustments in conditional probability; and for mathematical ease I've set my sample size at 221 beans for my typical 22 grams of coffee into the grinder for each espresso shot.
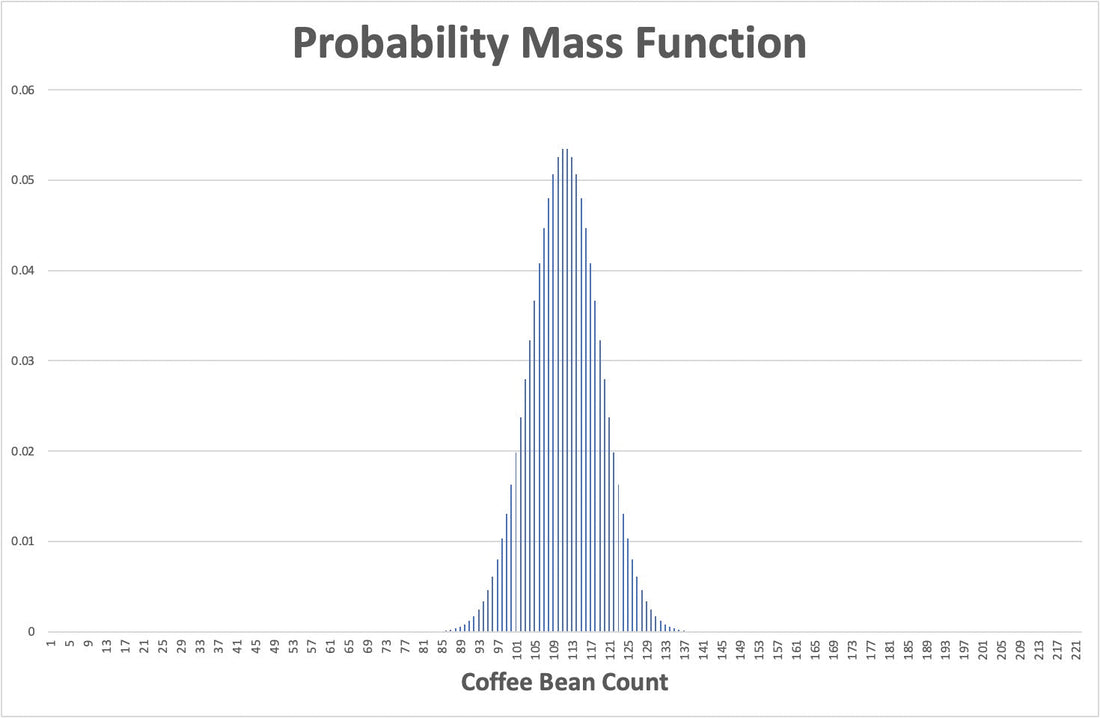
So imagine a 1 kg bag of this 50/50 blend. You have 5,000 beans of one coffee and 5,000 beans of another coffee. The binomial distribution is a statistical probability distribution that summarizes the likelihood that a value will take one of two independent values under a given set of parameters or assumptions (thank you Investopedia). We can therefore use the binomial distribution to model the probability mass function and look at the likelihood that a randomly selected set of beans for the morning espresso shot significantly deviates from the "ideal" 50/50 mix.
It turns out that two-thirds of the time your blend will not be worse than 53/47 and that 90% of the time the blend will not be worse than 56/44! I'm guessing that most coffee drinkers wouldn't be able to taste that difference and therefore I think that it's perfectly reasonable for coffee roasters to sell their blended coffee as blends rather than bag separate components which cause their customers to do a whole lot of unnecessary work!
